After working with students for 10 years, the same mistakes surfaced every time we introduced area and perimeter formulas for circles:
Messing up the order of operations. Take the traditional area formula, A=πr². Let’s say the radius is 3. Then students multiply π by 3, square that result, and get an incorrect answer.
Confusion over the meaning of π. “It’s a constant,” we tell them, or, “It’s just a number.” But consider: it’s a symbol, a Greek letter, that represents a ratio of two measures of a circle — in other words, it’s far from intuitive. It looks more like a variable than a number.
When calculating circumference, students will probably be successful since multiplying left to right works if we’re approximating π. But if we want students to give an exact answer in terms of π, they need to pluck π out of the middle of C=2πr, then take care of their multiplication, then throw π back on.
Let’s fix the formulas
What if we just put π at the end?

Now if you put a value in for r, just square it and you have an exact answer! The circumference formula gets the same treatment:
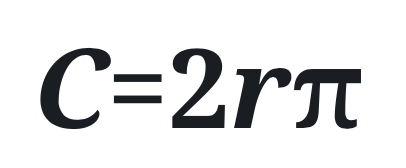
The only objection from teachers I’ve heard is this: But π is a number, and numbers are coefficients, and coefficients must go the left of the variable! Is it worth it to adhere to this convention if it creates confusion for students?
I say: Try it. See what your students think.
If removing ambiguity is an important principle of mathematics, then let’s teach students formulas that do just that.